#103: Santa's Symmetry Area Set

Uhhh, yes I know, it’s May. I made this set of symmetry area puzzles for a Secret Santa thing last year. I’ve been meaning to put it on the blog ever since, but I kept putting it off. Here it finally is.

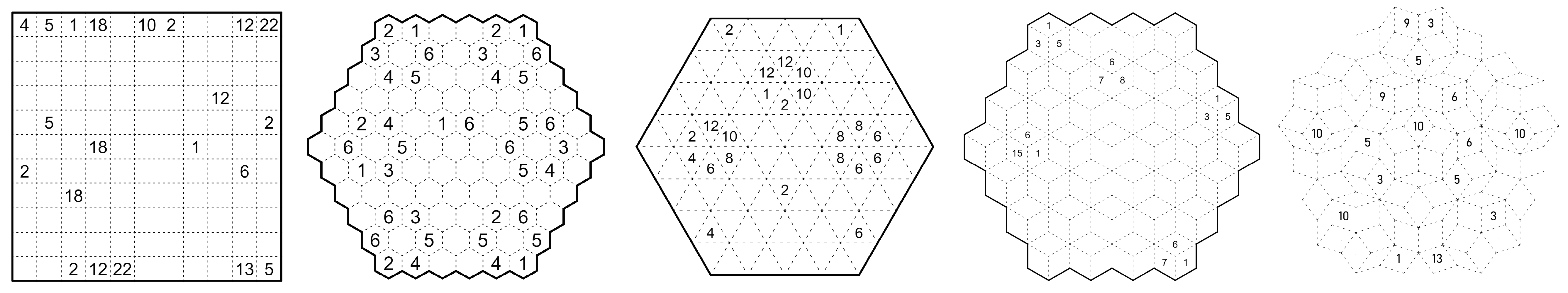
This is a pack of five challenging Symmetry Area puzzles on increasingly unusual grids. You can find online solving links for four of the five puzzles in this PDF (there’s no online solver for Penrose tilings, I’m afraid).

This was a gift for IHNN. They are one of the strongest solvers I know, so I wanted to make something decently tough to keep them occupied with the puzzles for more than a few minutes. Our main connection at the time was that we had both created something called “Hexagony” (an esolang in my case, and a puzzle competition in theirs), so that provided the seed idea for this gift.

The gimmick of their competition was that each genre had two puzzles, one of a square grid and one on a hex grid (but this was not known prior to the start of the contest… the rules were phrased generally enough to work for both). My idea for the gift was to up that a little and do one genre on several grids.

The original plan was making a Fillomino that had the same clue layout (shifted if necessary) on various grids, i.e. a weird multi-grid “doppel”. I did actually make that but it turned out extremely meh, so I scrapped it. Instead I decided to just make separate puzzles for each grid, which would allow me much more control over the logic to get the most out of each grid. I also switched to Symmetry Area, again to get more mileage out of the different geometries.

I hope you enjoy the puzzles! The hexagonal and triangular ones are the most approachable. The other three are all very difficulty. Note that for the rhombill tiling and the Penrose tiling, only the outline of each region must have rotational symmetry, not necessarily the cells inside them.

One last fun fact: I believe the 1-13 pair at the bottom of the Penrose grid is not actually required for uniqueness, which is just wild. They make the solve significantly less bashy though, so I kept them.